Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematics-Online course: Vector Calculus - Scalar Product

Scalar Product, Dot Product

[previous page] [next page] [table of contents][page overview]
The scalar product of two vectors is defined by
$\displaystyle \vec{a}\cdot\vec{b}$ $\displaystyle =$ $\displaystyle \vert\vec{a}\vert\vert\vec{b}\vert\cos\sphericalangle(\vec{a},\vec{b})$  
  $\displaystyle =$ $\displaystyle a_1 b_1 + a_2 b_2 + a_3 b_3 .$  

In particular, we have

$\displaystyle \vec{a}\cdot\vec{a} = \vert\vec{a}\vert^2 $

and

$\displaystyle \vec{a}\cdot\vec{b} = 0 \quad \Leftrightarrow \quad \vec{a} \perp \vec{b}\, . $

From the coordinate representation of the scalar product it follows

$\displaystyle \vec{a}\cdot\vec{b} = \vec{b}\cdot\vec{a} $

and

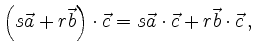
$\displaystyle \left(s\vec{a}+r\vec{b}\right)\cdot\vec{c} = s\vec{a}\cdot\vec{c}+r\vec{b}\cdot\vec{c}\, , $

i.e., the scalar product has properties analogous to some calculation rules of the product of real numbers.

(temporary unavailable)

(temporary unavailable)
[previous page] [next page] [table of contents][page overview]
  automatically generated 10/30/2007