Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematics-Online course: Linear Algebra - Matrices - Special Matrices

Fourier Matrices

[previous page] [next page] [table of contents][page overview]
Raising the root of unity

$\displaystyle w_n = \exp(2\pi\mathrm{i}/n) $

to higher powers we obtain the so called Fourier matrix

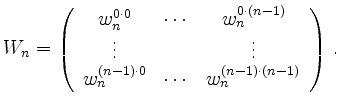
$\displaystyle W_n = \left(\begin{array}{ccc} w_n^{0\cdot 0} & \cdots & w_n^{0 ... ... w_n^{(n-1) \cdot 0} & \cdots & w_n^{(n-1)\cdot (n-1)} \end{array}\right)\, . $

Normalizing ( $ W_n \to W_n/\sqrt{n}$) yields a unitary matrix.
(Authors: App/Burkhardt/Höllig)

(temporary unavailable)

  automatically generated 4/21/2005