Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematics-Online lexicon: Annotation to

Tensor Products of Integration Rules

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z overview

Integration rules for rectangles

$\displaystyle Q = [a_1,b_1] \times \cdots \times [a_m,b_m] $

can be obtained by forming tensor products of univariate formulas.

\includegraphics[width=0.5\linewidth]{Bild_Rechteck_Quadratur}

If the formulas $ \sum_k w_{k,\nu} f(t_{k,\nu})$ for approximating $ \int_{a_\nu}^{b_\nu} f$ are exact for polynomials of degree $ \le n_\nu$, then the product rule

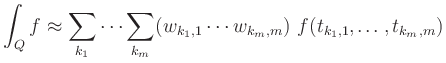
$\displaystyle \int_Q f \approx \sum_{k_1} \cdots \sum_{k_m} (w_{k_1,1}\cdots w_{k_m,m}) \ f(t_{k_1,1},\ldots,t_{k_m,m}) $

is exact for polynomials of coordinate degree $ \le (n_1,\ldots,n_m)$.

For simplicity, we consider functions of two variables ($ m=2$). To this end we denote by

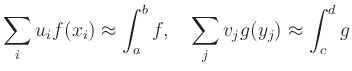
$\displaystyle \sum_i u_i f(x_i) \approx \int_a^b f,\quad \sum_j v_j g(y_j) \approx \int_c^d g $

the two univariate integration rules. Then, the bivariate formula has the form

$\displaystyle s = \sum_i u_i \left[ \sum_j v_j f(x_i,y_j) \right] \,. $

If $ f$ is a polynomial with coordinate degree $ \le (n_1,n_2)$,

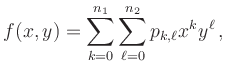
$\displaystyle f(x,y) = \sum_{k=0}^{n_1} \sum_{\ell=0}^{n_2} p_{k,\ell} x^k y^\ell \,, $

then, for fixed $ x_i$, the function $ f(x_i,y)$ is a polynomial of degree $ \le n_2$ in $ y$. Hence, the expression in brackets coincides with the integral $ \int_c^d f(x_i,y)\,dy$. Interchanging summation and integration, we obtain

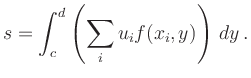
$\displaystyle s = \int_c^d \left( \sum_i u_i f(x_i,y) \right)\,dy \,. $

Again, the sum equals the corresponding integral, and it follows that

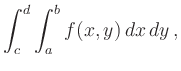
$\displaystyle \int_c^d \int_a^b f(x,y)\,dx\,dy \,, $

which is the anticipated value for $ s$.

[Back]
  automatisch erstellt am 17.  1. 2017