Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematics-Online lexicon:

Potential Functions, Gradient Fields and Line Integrals

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z overview
Let $ \Phi $ be a vector field and let $ u$ be a differentiable scalar function defined on a region $ D .$ Suppose that

$\displaystyle \Phi = \operatorname{grad} u \, . $

Then $ \Phi $ is called a gradient field and $ u$ is called a potential function of $ \Phi .$ The vector field $ \Phi $ is conservative, i.e. each line integral of $ \Phi $ in $ D$ is independent of the path.

More precisely let $ A$ and $ B$ be points in $ D .$ Let $ \sigma: [a,b] \longrightarrow D $ be a parametrization of a curve $ C$ in $ D$ beginning in $ \sigma (a) = A $ and ending with $ \sigma (b) = B .$ Then

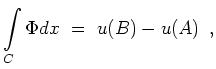
$\displaystyle \int\limits_{C} \Phi dx \ = \ u(B)-u(A) \ \,, $

i.e. the line integral is just the difference between the potentials at $ B$ and $ A .$

In particular for any closed path $ C$ in $ D$

$\displaystyle \int\limits_{C}\Phi \cdot dx \ = \ 0 .$

()

Examples:

[Annotations] [Links]
  automatically generated 6/15/2005