Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematics-Online lexicon:

Euclidean Normal Forms of Three-Dimensional Quadrics

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z overview
There exist appropriate Cartesian coordinate systems with respect to which the equations defining quadrics have the following normal forms.


conical quadrics


normal form name
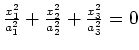$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}+\frac{x_3^2}{a_3^2}=0$ point
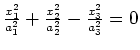$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}-\frac{x_3^2}{a_3^2}=0$ (double) cone
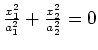$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}=0$ line
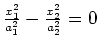$ \frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}=0$ intersecting planes
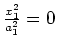$ \frac{x_1^2}{a_1^2}=0$ coincident planes


central quadrics


normal form name
$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}+\frac{x_3^2}{a_3^2}+1=0$ (empty set)
$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}-\frac{x_3^2}{a_3^2}+1=0$ hyperboloid of 2 sheets
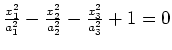$ \frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}-\frac{x_3^2}{a_3^2}+1=0$ hyperboloid of 1 sheet
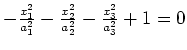$ -\frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}-\frac{x_3^2}{a_3^2}+1=0$ ellipsoid
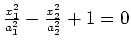$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}+1=0$ (empty set)
$ \frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}+1=0$ hyperbolic cylinder
$ -\frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}+1=0$ elliptic cylinder
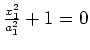$ \frac{x_1^2}{a_1^2}+1=0$ (empty set)
$ -\frac{x_1^2}{a_1^2}+1=0$ parallel planes


parabolic quadrics


normal form name
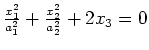$ \frac{x_1^2}{a_1^2}+\frac{x_2^2}{a_2^2}+2x_3=0$ elliptic paraboloid
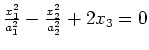$ \frac{x_1^2}{a_1^2}-\frac{x_2^2}{a_2^2}+2x_3=0$ hyperbolic paraboloid
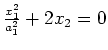$ \frac{x_1^2}{a_1^2}+2x_2=0$ parabolic cylinder


The normal forms are uniquely determined up to permutation of subscripts and in the case of conical quadrics up to multiplication by a constant $ c\ne 0$.

The values $ a_i$ are set to be positive and are called lengths of the principal axes of the quadric.

(double) cone intersecting planes
   
\includegraphics[width=.4\moimagesize]{quadriken_kegel} \includegraphics[width=.4\moimagesize]{quadriken_schneidende_ebenen}

hyperboloid of 2 sheets hyperboloid of 1 sheet
   
\includegraphics[width=.4\moimagesize]{quadriken_zweischaliges_hyperboloid} \includegraphics[width=.4\moimagesize]{quadriken_einschaliges_hyperboloid}

ellipsoid hyperbolic cylinder
   
\includegraphics[width=.4\moimagesize]{quadriken_ellipsoid} \includegraphics[width=.4\moimagesize]{quadriken_hyperbolischer_zylinder}

elliptic cylinder elliptic paraboloid
   
\includegraphics[width=.4\moimagesize]{quadriken_elliptischer_zylinder} \includegraphics[width=.4\moimagesize]{quadriken_paraboloid}

hyperbolic paraboloid parabolic cylinder
   
\includegraphics[width=.4\moimagesize]{quadriken_hyperbolisches_paraboloid} \includegraphics[width=.4\moimagesize]{quadriken_parabolischer_zylinder}

[Examples] [Links]
  automatically generated 7/13/2018