

Mathematics-Online lexicon:

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematics-Online lexicon: | ||
Multiple Integral | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
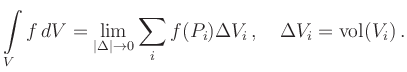
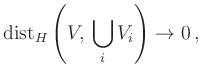
The notation
![]() symbolizes the approximation process,
and
symbolizes the approximation process,
and ![]() is called volume element.
A shorter notation is
is called volume element.
A shorter notation is
![]() , or, more detailed,
, or, more detailed,
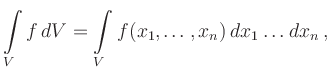
For ![]() multiple integrals are called double integrals, for
multiple integrals are called double integrals, for ![]() triple
integrals.
For double integrals one also uses the notation
triple
integrals.
For double integrals one also uses the notation
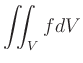 or
or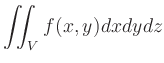
 or
or
Because of the continuity of the integrand ![]() , the definition of the multiple integral
is independent of the choice of the elementary volumes
, the definition of the multiple integral
is independent of the choice of the elementary volumes ![]() as well as of the points
as well as of the points
![]() .
.
![\includegraphics[width=0.5\moimagesize]{a_integral1}](/inhalt/aussage/aussage645/img20.png)
For a positive function, the integral coincides with the volume of the set
In order to garantuee the existence of multiple integrals,
weaker conditions on continuity and smoothness of ![]() and
and ![]() are possible.
The integral can also exist if the region of integration is unbounded.
Such an integral is called an improper integral.
are possible.
The integral can also exist if the region of integration is unbounded.
Such an integral is called an improper integral.
| automatically generated 9/22/2016 |