Mathematics-Online lexicon: Complex Resistance in A.C. Networks

	[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff]
	Mathematics-Online lexicon:
	Complex Resistance in A.C. Networks

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

overview

For the analysis of linear a.c. networks (alternating current network) it is advantageous to use polar coordinates. With the voltage

and the current

given as

$\displaystyle U(t) = U_0 e^{\mathrm{i}(\omega t+\varphi)}\,, \quad I(t) = I_0 e^{\mathrm{i}(\omega t+\psi)}\,,$

the complex resistance

$\displaystyle Z = U(t)/I(t)$

is independent of time. For the basic elements

resistor	coil	capacitor
$\includegraphics[width=.2\moimagesize]{komplexe_zahlen_schaltelement_widerstand.eps}$	$\includegraphics[width=.2\moimagesize]{komplexe_zahlen_schaltelement_induktivitaet.eps}$	$\includegraphics[width=.2\moimagesize]{komplexe_zahlen_schaltelement_kapazitaet.eps}$
	$Z=\mathrm{i}\omega L$	$Z=(\mathrm{i}\omega C)^{-1}$

the complex resistances are added when elements are serially connected:

$\displaystyle Z_{\text{total}} = Z_1 + Z_2$

On the other hand, when elements are connected in parallel, the resistance is calculated as the sum of their reciprocals:

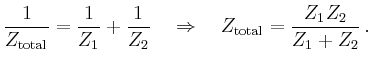
$\displaystyle \frac{1}{Z_{\text{total}}} = \frac{1}{Z_1} + \frac{1}{Z_2} \quad \Rightarrow \quad Z_{\text{total}}=\frac{Z_1 Z_2}{Z_1+Z_2}\, .$

$\operatorname{Re} Z$ is referred to as effective resistance, $\operatorname{Im} Z$ as reactance, and $\vert Z\vert$ as impedance.

$\includegraphics[width=.6\moimagesize]{komplexe_zahlen_schaltkreis.eps}$

As an example, the (total) resistance of the circuit, shown in the figure, is

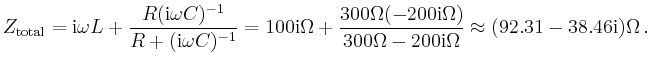
$\displaystyle Z_{\text{total}}=\mathrm{i}\omega L+ \frac{R(\mathrm {i}\omega C... ...a)}{300\Omega-200\mathrm {i}\Omega}\approx (92.31 -38.46\mathrm{i})\Omega \,.$

Moreover, with an a.c. voltage of $U_{\text{effective}}=220$ V,

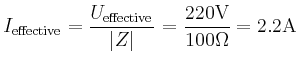
$\displaystyle I_{\text{effective}}=\frac{U_{\text{effective}}}{\vert Z\vert}=\frac{220\mathrm{V}}{100\Omega}=2.2\mathrm{A}$

is the effective current.

(Authors: Höllig/Wipper/Abele)

see also:

automatically generated 5/ 5/2011